Great Square supports Square Of your Binomial

Every time a binomial is normally squared, the effect we get is a trinomial. Squaring a binomial means, growing the binomial by itself. Reflect on we have a good simplest binomial "a plus b" and that we want to multiply the following binomial all alone. To show the multiplication the binomial could be written like the step below:

(a + b) (a +b) or (a + b)²

The above représentation can be carried out making use of the "FOIL" technique or making use of the perfect main square formula.

The FOIL process:

Let's make simpler the above copie using the FOIL method as explained down below:

(a plus b) (a +b)

sama dengan a² + ab & ba plus b²

sama dengan a² + ab plus ab + b² [Notice that ab sama dengan ba]

sama dengan a² & 2ab plus b² [As stomach + über = 2ab]

That is the "FOIL" method to fix the block of a binomial.

The Mixture Method:

Through formula approach the final reaction to the copie for (a + b) (a plus b) is normally memorized directly and employed it towards the similar problems. Why don't we explore the formula strategy to find the square of any binomial.

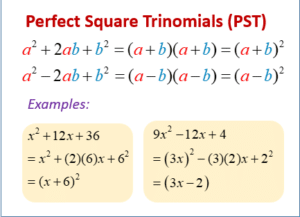
Invest in memory that (a + b)² sama dengan a² plus 2ab + b²

It really is memorized such as;

(first term)² + only two * (first term) 3. (second term) + (second term)²

Consider we have the binomial (3n + 5)²

To get the reply, square the first term "3n" which is "9n²", then simply add the "2* 3n * 5" which is "30n" and finally put the block of second term "5" which is "25". Writing this all in a step solves the square of the binomial. Discussing write it together;

(3n + 5)² = 9n² + 30n + 30

Which is (3n)² + only two * 3n * some + 5²

For example if you experience negative indication between the guy terms of the binomial then the second term will turn into the adverse as;

(a - b)² = a² - 2ab + b²

The given example can change to;

(3n - 5)² = 9n² - 30n + 24

Again, keep in mind the following to find square of a binomial immediately by the mixture;

(first term)² + two * (first term) (second term) + (second term)²

Examples: (2x + 3y)²

Solution: Primary term is definitely "2x" and the second term is "3y". Let's proceed with  perfect square trinomial  to carried out the square of this given binomial;

= (2x)² + 2 * (2x) * (3y) + (3y)²

= 4x² + 12xy + 9y²

If the indication is converted to negative, the treatment is still exact but replace the central indicator to unfavorable as revealed below:

(2x - 3y)²

= (2x)² + two * (2x) * (- 3y) & (-3y)²

sama dengan 4x² supports 12xy & 9y²

That could be all about developing a binomial by itself as well as to find the square of the binomial.